Generating Random Variables with given correlation

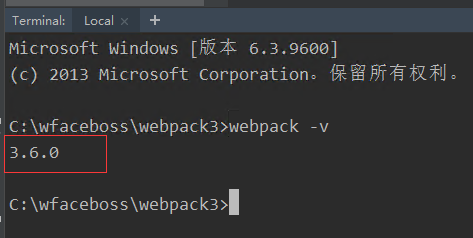
I want to generate 2 continuous random variables Q1, Q2 (quantitative traits, each are normal) and 2 binary random variables Z1, Z2 (binary traits) with given pairwise correlations between all possible pairs of them. Say

(Q1,Q2):0.23 
(Q1,Z1):0.55 
(Q1,Z2):0.45 
(Q2,Z1):0.4 
(Q2,Z2):0.5 
(Z1,Z2):0.47

Please help me generate such data in R.

This is crude but might get you started in the right direction.

library(copula)

options(digits=3)
probs <- c(0.5,0.5)
corrs <- c(0.23,0.55,0.45,0.4,0.5,0.47)  ## lower triangle

Simulate correlated values (first two quantitative, last two transformed to binary)

sim <- function(n,probs,corrs) {
    tmp <- normalCopula( corrs, dim=4 , "un")
    getSigma(tmp) ## test
    x <- rCopula(1000, tmp)
    x2 <- x
    x2[,3:4] <- qbinom(x[,3:4],size=1,prob=rep(probs,each=nrow(x)))
    x2
}

Test SSQ distance between observed and target correlations:

objfun <- function(corrs,targetcorrs,probs,n=1000) {
    cc <- try(cor(sim(n,probs,corrs)),silent=TRUE)
    if (is(cc,"try-error")) return(NA)
    sum((cc[lower.tri(cc)]-targetcorrs)^2)
}

See how bad things are when input corrs=target:

cc0 <- cor(sim(1000,probs=probs,corrs=corrs))
cc0[lower.tri(cc0)]
corrs
objfun(corrs,corrs,probs=probs) ## 0.112

Now try to optimize.

opt1 <- optim(fn=objfun,
              par=corrs,
              targetcorrs=corrs,probs=c(0.5,0.5))
opt1$value     ## 0.0208

Stops after 501 iterations with "max iterations exceeded". This will never work really well because we're trying to use a deterministic hill-climbing algorithm on a stochastic objective function ...

cc1 <- cor(sim(1000,probs=c(0.5,0.5),corrs=opt1$par))
cc1[lower.tri(cc1)]
corrs

Maybe try simulated annealing?

opt2 <- optim(fn=objfun,
              par=corrs,
              targetcorrs=corrs,probs=c(0.5,0.5),
              method="SANN")

It doesn't seem to do much better than the previous value. Two possible problems (left as an exercise for the reader are) (1) we have specified a set of correlations that are not feasible with the marginal distributions we have chosen, or (2) the error in the objective function surface is getting in the way -- to do better we would have to average over more replicates (i.e. increase n).