Transposing a 2 dimensional matrix in Erlang

问题:

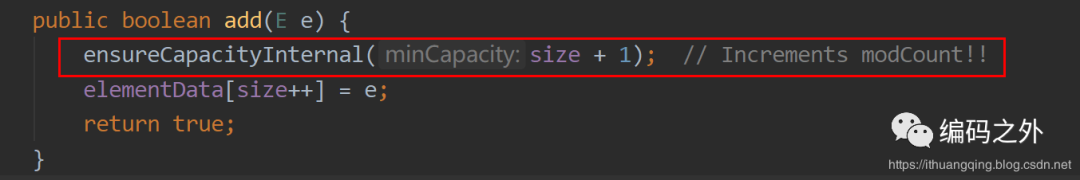
Given a matrix like below, transform it, say, 90 degrees into the second matrix below. How would you go about doing this in the cleanest way possible? Short/succinct/clear solutions where the point is easy to grasp is preferred.

From

[[A1,A2,A3],
 [B1,B2,B3],
 [C1,C2,C3]]

To

[[A1,B1,C1],
 [A2,B2,C2],
 [A3,B3,C3]]

Edit: I realize it was not clear from original question. I'd like to know how to do this in Erlang.

回答1:

Simplifying the solutions already given, you can do it in as short as:

-module(transp).

-export([transpose/1]).

transpose([[]|_]) -> [];
transpose(M) ->
  [lists:map(fun hd/1, M) | transpose(lists:map(fun tl/1, M))].

回答2:

In functional programming languages, the usual approach for matrix transposition is to use unzip.

回答3:

Here's my sample solution:

-module(transp).

-export([transpose/1]).

transpose(L) ->
     transpose_do([], L).

transpose_do(Acc, [[]|_]) ->
     lists:reverse(Acc);
transpose_do(Acc, M) ->
     Row = lists:foldr(
          fun(Elem, FoldAcc) ->
                    [hd(Elem) | FoldAcc]
          end,
          [],
          M),
     transpose_do([Row|Acc], lists:map(fun(X) -> tl(X) end, M)).

Test:

1> M = [[a1,a2,a3],[b1,b2,b3],[c1,c2,c3]].
[[a1,a2,a3],[b1,b2,b3],[c1,c2,c3]]
2> transp:transpose(M).   
[[a1,b1,c1],[a2,b2,c2],[a3,b3,c3]]

回答4:

Here's an implementation that I think I got from the Haskell standard library:

%% Transpose rows and columns in a list of lists. Works even if sublists
%% are not of same length. Empty sublists are stripped.
transpose([[X | Xs] | Xss]) ->
    [[X | [H || [H | _] <- Xss]]
     | transpose([Xs | [T || [_ | T] <- Xss]])];
transpose([[] | Xss]) -> transpose(Xss);
transpose([]) -> [].

Compact and slightly mind-bending.

回答5:

What you are showing is not a matrix rotation, but rather matrix transposition. If you call the first matrix A and the second B then you have

A[i,j] = B[j,i]

To go from A to B you just need two nested loops with i = 1 to n and j = i+1 to n and at each iteration you swap the off-diagonal entries using a temporary variable.